NPTEL Computer Vision Week 2 Assignment Answers 2024
1. A possible equivalent representation of a 2D point (1, 3) in 𝑅2 after projecting onto 𝑃2 is
a) (3, 6, 3)
b) (6, 18, 6)
c) (4, 16, 4)
d) (6, 12, 6)
Answer :- Click Here
2. Find the transformation of the line 2𝑥 − 5𝑦 + 3 = 0.
a) 2𝑥 − 𝑦 − 4 = 0
b) 4𝑥 − 1.5𝑦 − 4.5 = 0
c) 4𝑥 + 𝑦 − 5 = 0
d) 2𝑥 − 0.5𝑦 + 4.5 = 0
Answer :-
3. Find the transformation of the point 𝑝(2, 0, 1).
a) 𝑝(4, 3, 1)
b) 𝑝(2, 1.5, 1)
c) 𝑝(2, 3, 1)
d) 𝑝(3, 4, 1)
Answer :-
4. Compute the line passing through the points (3, 2) and (6, 0).
a) −3𝑥 + 2𝑦 + 1 = 0
b) −2𝑥 + 5𝑦 + 12 = 0
c) −5𝑥 − 2𝑦 + 1 = 0
d) 2𝑥 + 3𝑦 − 12 = 0
Answer :- Click Here
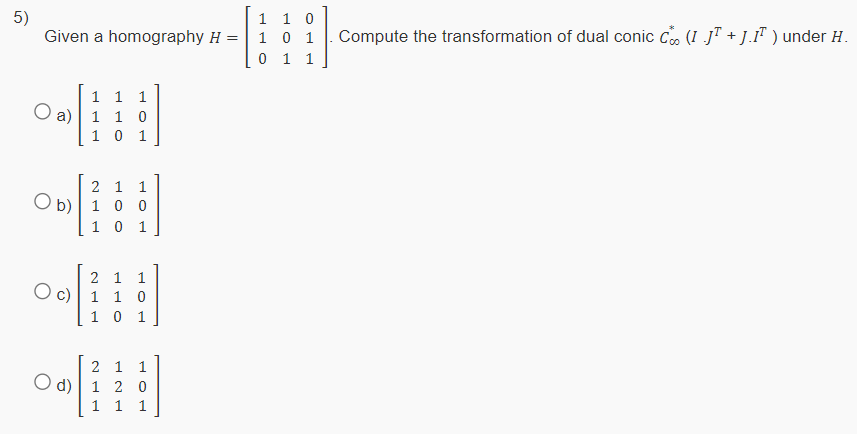
Answer :-
Answer :- Click Here
7. Which of the following statements are true?
a) The degree of freedom for similarity group is 4 (1 scale, 2 rotation, 1 translation).
b) The circular points are fixed points under the projective transformation 𝐻 if and only if 𝐻 is a similarity.
c) A conic remains a conic under homography.
d) Any square matrix can be decomposed as a product of an orthogonal matrix and an upper triangular matrix.
Answer :-
8. Given two lines 𝑙 = 2𝑥 + 𝑦 − 3 = 0 and 𝑚 = 3𝑥 − 2𝑦 + 1 = 0 meet at a point 𝑝. Find the Euclidean angle between these two lines. Answer should be in nearest degrees. Discard the decimal values.
Answer :-
Answer :-
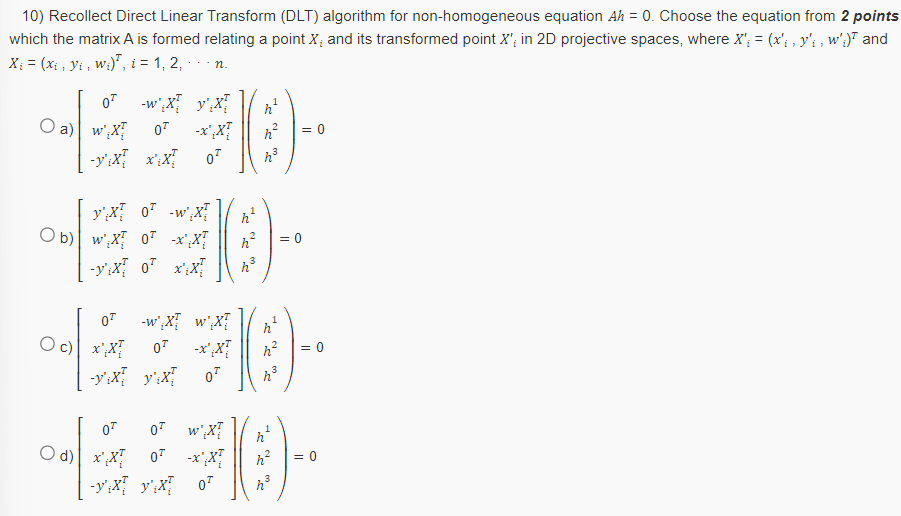
Answer :- Click Here
![[Week 3] NPTEL Computer Graphics Assignment Answers 2023](https://dbcitanagar.com/wp-content/uploads/Computer-Graphics-150x150.png "NPTEL Computer Graphics Week 2 Assignment Answers 2024 6")
